Historical Astronomy: Concepts: Triangulating an Orbit

Once you know the details of the earth's orbit, and know the position of the earth for any date, it is pretty easy to use triangulation to plot the orbit of any planet. In order to triangulate a position to a planet, however, you need a large baseline because the planets are pretty far away. Even making observations from opposite sides of the earth is not enough of a baseline. (Technically, with modern telescopes, that would be fine, but we are trying to get into the spirit of Kepler's problem.)

Imagine that you go out tonight and find the position for a planet (say Mars.) If you wait a couple days and look at Mars, it will appear to be in a different position in the sky for two reasons: the earth is moving and Mars is moving. However, if you wait 687 days instead, then Mars will be in the exact same place in its orbit, but, from the earth, it will appear to be in a different position because of the motion of the earth. (The sidereal period of Mars is 687 days.) In order to triangulate the position of a planet, all we need to really know is the sidereal period of the planet. In the example of Mars, since we know it takes Mars 687 days to go around the sun once, every 687 days, Mars is in the same spot. To triangulate the position of Mars, just use two sightings that are 687 days apart; Mars will be in the same spot, but the earth will not, and so Mars will appear to be in a very different place because of the earth's motion. SInce we know exactly where the earth is on any given day, then we can use those sightings to triangulate.

Here's a more concrete example. Let's say that we go out tonight, March 21, and observe that Mars is at a position of 118.5 along the ecliptic. 687 days later, on Feb 5, we see that Mars appears to be at a position of 169 along the ecliptic. Because it is 687 days later, Mars is actually in the same spot, but the earth moved, so it looks like Mars has moved because of parallax. If we find the position of the earth on those two days, and then triangulate from them, we get something that looks like the picture below (The triangulation lines are the red lines, and the orbit of the earth is the blue circle.):

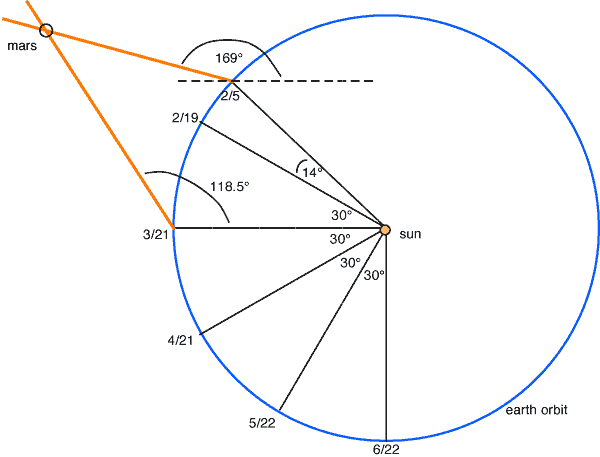
All we have to do is repeat what we did earlier; find the position of the earth, and draw another sighting line. The two lines intersect at the position of Mars at that point in its orbit. To fully figure out the orbit of Mars, we just use whole lot of pairs of observations. In each pair of dates, we make sure they are exactly 687 days apart. We also make sure that the pairs are spread out in time so that we are looking at Mars at different points in its orbit.

The data below is made up, but will give an accurate plot of the orbit of Mars. It has eight pairs of sightings for eight different spots of the orbit of Mars.

Position	Date	Direction to Mars
1	Mar. 21, 1931	118.5
	Feb. 5, 1933	169
2	Apr. 20, 1933	151.5
	Mar. 8, 1935	204.5
3	May 26, 1935	187
	Apr. 12, 1937	245.5
4	Sept. 16, 1939	297
	Aug. 4, 1941	16.5
5	Nov. 22, 1941	12
	Oct. 11, 1943	80
6	Jan. 21, 1944	65.5
	Dec. 9, 1945	123
7	Mar. 19, 1946	107.5
	Feb. 3, 1948	153
8	Apr. 4, 1948	138
	Feb. 21, 1950	190.5

last updated 3/6/08 by david mcclung, copyright 2003. All rights reserved.