Historical Astronomy: Concepts: Size of the Earth

To measure the size of the earth, you only need to know a couple things: the distance between two places on the earth, and their angular separation. The ancient Greeks were able to figure this out, and Eratosthenes made a pretty accurate measurement of the size of the earth.

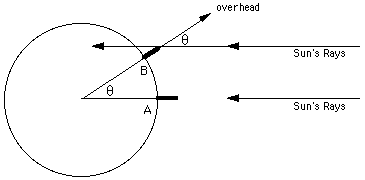
It is pretty easy to measure the angular distance between two places, as long as careful about the places to choose. Assume there is a town (A) at which, at some point in the year, the sun is directly overhead. (This happens for any place between the Tropics of Cancer and Capricorn.) If the sun is directly overhead, then a stick that is stuck straight in the ground will not cast a shadow. While this is happening, at some other town (B) the sun is not directly overhead, so that a stick will cast a shadow on the ground. (See diagram below.)

In diagram above, the sun is directly overhead of town A, but not directly overhead in town B. The angle is the angular separation between the towns. At town B, a stick of length L is stuck in the ground so that it is pointing straight up. (See diagram at right.) Because the sun is not overhead in B, the stick has a shadow of length d. From the diagrams, it should be obivous that

Tan = d/L

The ancient Greeks would have paced out the distance D between the towns. Using the above to calculate , and a little geometry, we can say

/360 = D/C

C is then the circumference of the earth.

last updated 6/3/03 by david mcclung, copyright 2003. All rights reserved.